How salty does the sea have to be for an egg to float?
Try this eggs-periment to learn why some objects float in the ocean while others sink

Which glass holds the salty water? This activity will help you figure it out.
Arthit Pornpikanet/iStock/Getty Images Plus
Summary
Objective: Determine what salt concentration will float an egg
Areas of science: Ocean sciences
Difficulty: Intermediate/Easy
Time required: ≤ 1 day
Prerequisites: None
Material availability: Readily available
Cost: Very low (under $20)
Safety: Always wash your hands after handling uncooked eggs because they may carry Salmonella.
Credits: Andrew Olson, PhD, Science Buddies; Sandra Slutz, PhD, Science Buddies
Did you know that if you put an egg in a cup of tap water, it will sink to the bottom? But, if you add enough salt, the egg will float back up to the surface! Why? Because the density of the egg is higher than the density of tap water, so it sinks.
Density (ρ), as shown in Equation 1, is the mass (m) of a material per unit volume (v). For example, the density of freshwater under standard conditions is approximately 1 gram (g) per cubic centimeter (cm3). In other words, if you filled a 1-cm x 1-cm x 1-cm box with freshwater, the water inside the box would have a mass of 1 g.
Adding salt to the water increases the density of the water, because the salt increases the mass without changing the volume very much. With enough added salt, the saltwater solution density is higher than the egg’s, and the egg will then float, as shown in Figure 1. The ability of something, like the egg, to float in water or some other liquid is known as buoyancy.
Equation 1:
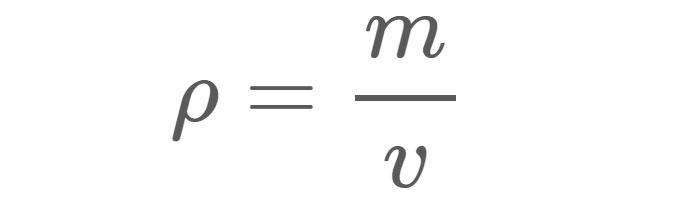
ρ = Density in whatever units are used for mass and volume.
m = Mass in grams (g), kilograms (kg), or any other unit of weight.
v = Volume in centimeters cubed (cm3), meters cubed (m3), or any other unit of volume.
But just how much salt is needed to make an egg float? In this science fair project, you will figure that out by placing an egg in cups with different salt concentrations. The concentration of a solution tells you how much of a compound is in a certain volume of a mixture.
In chemistry, the mass concentration is one way of expressing the concentration of a solution. The mass concentration is defined as the mass of a compound (in grams) in a certain solvent volume (in liters) and has the unit grams per liter (g/L). For example, in a solution with 750 grams of salt (sodium chloride or NaCl) in 1.5 liters of water, the mass concentration of salt is 750 g/1.5 L = 500 g/L.
In this project, you will be using the technique of making serial dilutions to create solutions with different salt concentrations. A serial dilution is a method for accurately diluting a solution in regular steps. You add a known amount of your starting, or stock, solution to a known amount of water and mix them. This process is called dilution. Diluting a solution means adding additional solvent (water in this project) to decrease the solution’s concentration. The new concentration of the diluted solution can be calculated using Equation 2.
Equation 2:
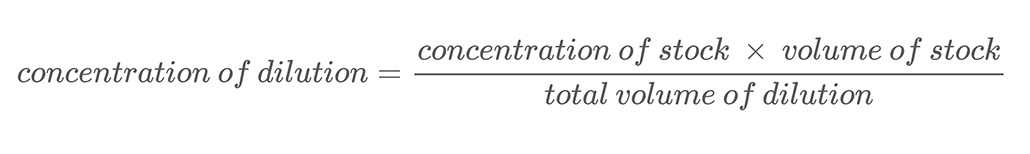
Here is an example calculation. Let’s say you have a salt solution with a mass concentration of 500 g/L. You dilute this solution by mixing 0.25 L of that salt solution with 0.25 L of water. This brings the total volume of your dilution to 0.5 liters (0.25 L + 0.25 L). To calculate the mass concentration of salt in the diluted salt solution you use Equation 2:
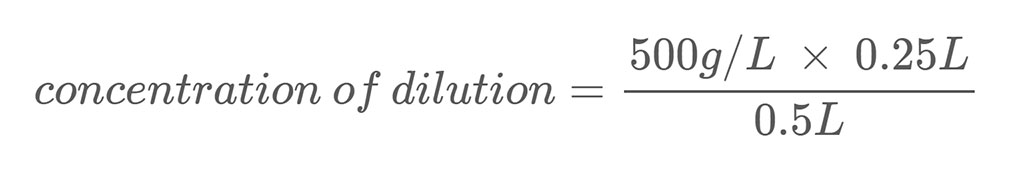
Solving the equation tells you that your dilution has a salt concentration of 250 g/L, which is half of your stock solution.
As a general rule, if the volume of stock solution and the volume of solvent (water) for your dilution are equal, you will be diluting the solution by half. This is called a two-fold dilution. A two-fold dilution means that with each dilution step, the new concentration of the dilution should be 50 percent of the original concentration.
If you want bigger steps, you should use relatively more water; if you want smaller steps, you should use relatively less water. By repeating the process, you can make a whole series of dilutions, which is how the method got its name. In this ocean science project, you will start out using two-fold dilutions to find out how much salt will cause an egg to float.
Terms and concepts
- Density
- Mass
- Volume
- Buoyancy
- Serial dilution
- Stock
- Mass concentration
- Relative concentration
- Absolute concentration
Questions
- Why would an egg float in water with a lot of salt in it, but not in plain tap water?
- What happens to salt (sodium chloride or NaCl) molecules when dissolved in water?
- Why does adding salt to water increase its density?
Materials and equipment
- Eggs (5)
- Permanent marker
- Table salt (1 cup)
- Water
- Measuring cup, liquid
- Large container, such as a large bowl or cooking pot. Must be able to hold at least five cups.
- Spoon for stirring
- Bag of clear 16-oz plastic cups
- Soup spoon for egg transfer
- Lab notebook
Experimental Procedure
- Note: For convenience of doing this science project using household measuring tools, volumes are given in terms of United States measuring spoons and cups. However, science is done in metric units and students may need to convert when writing up their procedure. To convert units, you can use the following website:
- Science Made Simple, Inc. (n.d.). Metric conversions & US customary unit conversion calculator. Retrieved April 15, 2013.
- Take five eggs out of the refrigerator, use a permanent marker to label them 1-5, and allow them to warm to room temperature.
- Make a stock solution of 1 cup of salt dissolved in 5 cups of water, as follows:
- Pour 3 cups of water into your large container.
- Add 1 cup of salt.
- Stir to dissolve some of the salt. It will not all dissolve yet.
- Add 2 more cups of water.
- Stir to dissolve the rest of the salt. The salt should be completely dissolved before you go on to the next step.
- This may take several (5 to 10) minutes of stirring, so you may need to be patient.
- This may take several (5 to 10) minutes of stirring, so you may need to be patient.
- Make a two-fold serial dilution of the stock solution, as follows:
- Label five of the plastic cups 1-5. Cup 1 will be for the stock solution, cups 2-4 will be for the dilutions, and Cup 5 will be plain tap water.
- Add 3/4 cup of your stock salt solution to Cup 1.
- Add 3/4 cup plain tap water to cups 2-5.
- Measure out 3/4 cup stock solution, and add it to Cup 2. Mix.
- Measure out 3/4 cup of the solution from Cup 2 and add it to Cup 3. Mix.
- Measure out 3/4 cup of the solution from Cup 3 and add it to Cup 4. Mix.
- What are the absolute mass concentrations of salt in cups 1-4? (To calculate with metric units, use these conversions: 1 cup of salt is 292 grams [g], 1 cup of water is 237 milliliters [mL], and 3/4 cup of stock solution is 177.75 milliliters [mL]). Write these concentrations down in your lab notebook. Review the Introduction section if you need help with your calculations.
- What are the relative salt concentrations in cups 2-4 compared to the original stock solution? Use the absolute mass concentrations that you calculated in the previous step for your calculations. Example: Let’s assume that the original stock solution in Cup 1 has a salt concentration of 500 g/L. Cup 3 has a salt concentration of 125 g/L. The relative salt concentration can be calculated as the ratio of 125 g/L / 500 g/L, which is 0.25. Expressed as a percentage, this would be 25%. Therefore, Cup 3 has a relative salt concentration of 25% compared to Cup 1.
- Now, starting with Cup 5 and working your way up, test an egg in each solution to see if it will float. Use a soup spoon to lift the egg in and out of the cups.
- In which cup did the egg first float? (Save this solution for step 7.) If the egg floated in more than one cup, did you notice any difference in how it floated?
- Be sure to record your results and observations in your lab notebook, including the egg’s number.
- Be sure to record your results and observations in your lab notebook, including the egg’s number.
- Repeat steps 5-6 with four other eggs.
- Now you know, within a factor of 2, how much salt it takes to float an egg. How can you narrow down the range further to get a more precise estimate? By doing another serial dilution, of course.
- This time you will start your dilution with the salt concentration in which the egg first floated, the one you selected in step 6.
- Figure out a new serial dilution with smaller steps. For example, you could try diluting the solution by 25 percent with each step. That means with each step, the new concentration should be 75 percent of the original concentration.
- What amounts of stock solution and water do you need to use?
- Remember that you will need enough solution to more than cover the egg, which will probably be around 3/4 cup, and you probably cannot fit more than 2 cups of solution in each 16-oz cup.
- Hint: You may only be able to test the first few cups in a dilution series at a time unless you use larger cups.
- Tip: If you need additional help for making serial dilutions, check out the serial dilutions resource in the Bibliography in the Background section.
- Write up your new dilution procedure in your lab notebook, including the calculated relative and absolute salt concentrations for each cup.
- Make the new dilution series. Remember to start with the salt concentration where the egg first floated. (If you do not have enough solution from the original serial dilution, make some more by starting from the stock solution.)
- As before, test an egg in each cup, starting with the lowest salt concentration. In which cup did the egg float first?
- Be sure to record your results and observations in your lab notebook, including the egg’s number.
- Repeat this step with the four other eggs.
- If you want, make another dilution series, with even smaller steps, to improve the precision of your estimate.
- Be sure to record your results and observations in your lab notebook, including the egg’s number.
- Repeat this step with the four other eggs.
- When you are done handling the eggs, wash your hands with soap and warm water. It is important to wash your hands after handling uncooked eggs because they may carry Salmonella.
- Determine the densities for all five eggs and record this in your lab notebook.
- Hint: If the density of the saltwater is less than the egg’s density, the egg will sink, and if the density of the saltwater is greater than the egg’s density, the egg will float. So the density of the egg would be between these two absolute salt densities.
- Hint: If the density of the saltwater is less than the egg’s density, the egg will sink, and if the density of the saltwater is greater than the egg’s density, the egg will float. So the density of the egg would be between these two absolute salt densities.
- Plot the densities for all five eggs on a chart, putting the egg’s number on the x-axis and its density on the y-axis. What is the density of the eggs? How much variation in density is there from egg to egg?
Variations
- Does a hard-boiled egg float at the same salt concentration as an uncooked one? Hint: You will need to measure the same egg before and after hard boiling and be very precise about your serial dilutions.
- Find out how much salt there is in sea water. From the results of your experiment, predict whether an egg would float or sink in sea water. (If you live close enough to the ocean, you can get collect some sea water and test your prediction!)
- Figure out another method of determining the density of an egg. Compare the density measurements for the same eggs using your method and this salt water float test.
This activity is brought to you in partnership with Science Buddies. Find the original activity on the Science Buddies website.








