Teen puts calculus on ice
Jacob Nichols calculated the volume of long, skinny icicles using advanced math
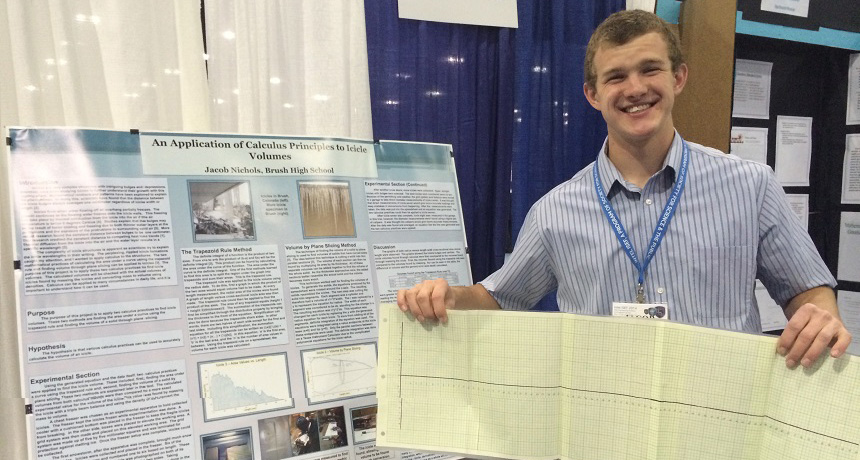
Jacob Nichols decided to use calculus to find the volumes of the icicles around his house.
B. Brookshire/SSP
LOS ANGELES — Jacob Nichols, 19, points to a picture of his house in Brush, Colo. The roof is festooned with huge icicles. “It’s pretty cold in the winter, and we get a lot of snow,” he explains. So when the recent Brush High School graduate started looking for a science fair project, the answer was right outside his window. He would see if he could apply the principles of calculus to icicles.
His findings earned him a spot at the 2014 International Science and Engineering Fair. The annual fair, created by Society for Science & the Public and sponsored by Intel, brings high school students from around the world together to connect and show off their science projects. (SSP also publishes Science News for Students and this blog).
You can easily figure out the volume of an icicle. Just take the mass of your ice in grams, divide it by the known density of ice (0.9 grams per cubic centimeter), and you end up with the volume in cubic centimeters. But this doesn’t take the shape of the ice into account. Jacob wanted to know if you could apply calculus to a long, skinny icicle and find the volume.
He started with the most entertaining part: collecting the icicles. “I got the longest icicles I could find from all over town,” he recalls. One was well over 1.5 meters (5 feet) long, but unfortunately proved to be no good for his experiments. He took the best icicles and stored them in his freezer.
Then, during an extra cold snap, Jacob carefully took each icicle into his unheated garage and measured it out on graph paper. “I took three icicles and placed them on this grid system I made,” he explains. “I measured the diameter every five millimeters to get as many measurements as possible. And then I rotated the icicles 90 degrees and measured the diameter again to take out error. I averaged those two measurements to find the average radius of the icicle at each five-millimeter point.”
Those measurements became the teen’s data points. He then attacked the problem using two calculus methods. One was the trapezoid rule. This method divides a long curve (like the subtle curve in a tapering icicle) into tiny trapezoids, the five-millimeter segments that Jacob measured. By finding the area of each trapezoid, he could calculate the area under the long icicle curve. Then, he could use that area calculation combined with the radii data that he gathered to calculate the volume of the icicle.
Jacob checked his work with a second method called the plane slicing method. This method takes all of the data points that Jacob measured and produces a line of that best shows which direction the points are going. The change in the line, calculated with the radius of the icicle, produces the volume.
The teen compared both of his methods against the usual method for calculating volume, weighing his three icicles and multiplying by ice’s density. His two calculus methods were each slightly off, but not by much. Jacob says that he “probably could have rotated the icicle a few more times” to get more measurements that would make his final volume more accurate.
“I didn’t know of anyone who had tried to find the volume of icicles this way,” he explains, “and that’s what really intrigued me.” Jacob found a new way to use calculus, just from observing the world outside his window.
Follow Eureka! Lab on Twitter
Power Words
calculus Using math to measure change. Calculus can be applied to measure change if that change can be plotted on a curved line. Differential calculus works with the rates of change in the slopes of curves, while integral calculus is concerned with the areas underneath curves.
density A measure of the consistency of an object, found by dividing the mass by the volume.
integration A fundamental principle of calculus that can be used to determine the area under a curve.
volume The quantity of space enclosed by a boundary. This can be a physical boundary such as a cup which holds a volume of water, or a boundary between one type of substance and another. For example, an ice cube floating in water has a specific volume.







