Wired for Math
Our brains seem to come equipped with systems for estimating amounts and adding numbers.
By Emily Sohn
Quick. What’s 845 + 289? Sorry, time’s up.
Few people can add numbers in their heads that quickly. If someone showed you a set of 845 dots next to a set of 289 dots, however, you’d probably be able to tell right away which set has more dots. You could probably also tell that 845 dots is more than 100 dots plus 189 dots, even if you saw all three sets of dots separately.
 |
|
How quickly can you solve this problem? |
By giving tests like these to kids and adults, scientists are discovering that we develop a sense for large numbers long before we learn how to count or talk. Our brains seem to come equipped with systems for estimating amounts and doing arithmetic, says Elizabeth Spelke. She’s a psychologist at Harvard University in Cambridge, Mass.
Spelke’s work adds to a growing body of research showing that young children and even animals have some inborn sense of number, long before they ever receive a formal math lesson (see “It’s a Math World for Animals“).
Spelke’s goal is to answer one basic question. “What abilities do children have when they start school that support the learning of mathematics?” she asks.
The answer, Spelke says, could help teachers find better ways to teach math by tapping into skills that their students bring with them.
 |
|
Learning what skills children already have when they start school could help teachers find better ways to teach math. |
| Artville |
“The premise of my work is that all learning in school builds on systems we already have,” Spelke says. “My goal is to figure out what those systems are, how they work, and how they come together for mathematical thinking.”
Flash math
To add, subtract, multiply, or divide, you need to understand that numbers are symbols that can be used in a variety of settings. The symbol 11, for instance, can refer to your age, the number of days left before vacation, the number of chimes you hear when the clock strikes 11 a.m., or the total number of cookies left over in three different boxes.
That’s a tricky concept, and the ability to understand numbers as symbols is one thing that separates people from other animals. Unlike other animals, people can figure out exactly what amount you get when you add 32 and 16, without even having to know whether the numbers refer to coins, apples, or anything else.
Spelke and her coworkers have done various experiments to find out how well young children can estimate quantities and add large numbers—even before they’re taught arithmetic in school.
Their most recent attempt to uncover the foundations of our mathematical abilities involved five experiments. In each experiment, preschoolers sat in front of computers watching animations that briefly flashed various sets of dots on the screen. Each set contained from 10 to 58 dots.
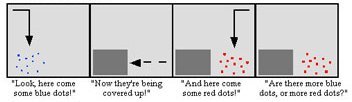 |
|
In the first experiment, children saw a set of blue dots, then a set of red dots on a computer screen. They had to decide whether there were more blue dots or red dots. |
| Proceedings of the National Academy of Sciences (PNAS) |
First, Spelke and her colleagues presented a group of 5-year-olds with one set of blue dots and one set of red dots. The sets of dots appeared one at a time, and they flashed by too quickly to be counted.
The results showed that the children were usually able to pick the larger set, even if the dots were different sizes and took up different amounts of space.
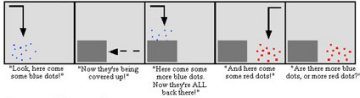 |
|
In the second experiment, five-year-olds had to decide whether the total number of blue dots in two sets was larger or smaller than the number of red dots. |
| Proceedings of the National Academy of Sciences (PNAS) |
For the second experiment, the 5-year-olds saw one group of blue dots, which was followed by a second group of blue dots, which was followed by a group of red dots. This setup was more complicated than the first, but the kids were still able to say whether there were more blue dots altogether or red dots, suggesting that they could both add and compare amounts.
In the third experiment, the children had to compare a group of dots that they saw to a certain number of tones that they heard. They could still pick the larger group. In the fourth, they successfully chose the largest grouping, given two sets of dots of the same color and one set of tones.
 |
|
In the third experiment, young children briefly saw a set of blue dots, then heard a certain number of tones (one tone for each red dot). They had to decide whether there were more blue dots or tones (red dots). |
| Proceedings of the National Academy of Sciences (PNAS) |
These results suggest that kids have an ability to add and compare numbers in different settings.
Word problems
In the fifth set of experiments, the researchers used words instead of dots or tones to present comparison problems.
Here’s an example that’s like the second experiment with just dots. Suppose you have 15 pennies. Your Mom gives you about 10 more. Your sister has about 40. Who has more pennies: you or your sister?
When given in words, the 5-year-olds couldn’t answer the question correctly. Most of them, however, could answer the question when it was given as sets of dots.
Overall, Spelke concluded that a child’s sense of number doesn’t depend on his or her ability to use language. Other studies with adults, she says, have shown that we use one part of our brains when doing arithmetic, such as adding 43 plus 72, and another part when estimating and comparing sets of dots.
Building blocks
So, certain building blocks for learning math seem to be in place before kids start school, Spelke says. It then takes years of math class for the brain to learn how to combine its inborn number sense with its language and other symbolic skills. High grades on algebra tests are the final result.
 |
|
Learning elementary arithmetic is surprisingly hard for lots of kids. |
If you’re trying hard and still getting B’s and C’s in math, though, you’re not alone.
“Learning elementary arithmetic is surprisingly hard for all children,” Spelke says. Her research shows, however, that you might be better at certain types of math than you think you are. That should give you hope.
“It is both surprising and encouraging to see,” Spelke says, “that if you engage kids in thinking about number outside of symbolism, they understand a lot.”
Even then, most kids need to take classes and do lots of homework to learn arithmetic.
The effort is worth it, Spelke says, because without math, our culture couldn’t function. “So much of our understanding of the world depends on mathematics,” she says.
“Math is fundamental to measurement,” Spelke says. “It’s fundamental to technology. It’s fundamental to science.”
Going Deeper:







