Questions for ‘Cool Jobs: Making electronics to wear’
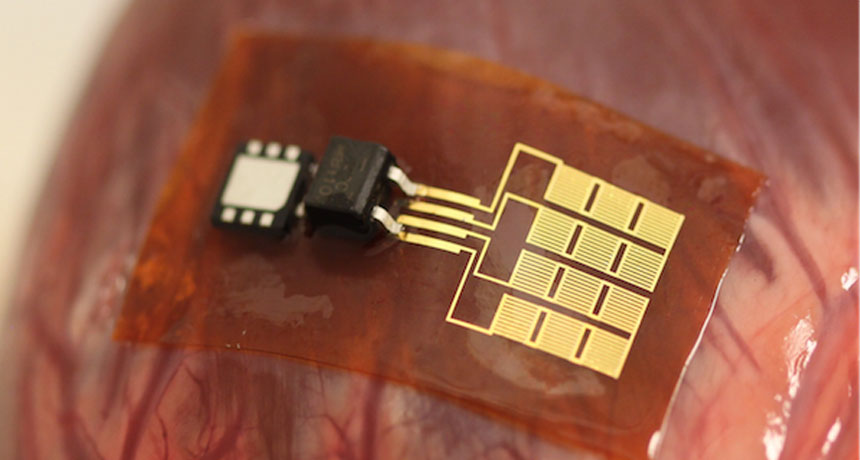
This electronic device has been attached to a heart so that it can use the beating movements as a source of energy to power some device.
Canan Dagdevirin
Come explore with us!

This electronic device has been attached to a heart so that it can use the beating movements as a source of energy to power some device.
Canan Dagdevirin
To accompany feature ‘Cool Jobs: Making electronics to wear’
1. What new things do you think a smartphone could do if the technology inside it were flexible instead of stiff?
2. What about if that technology also was waterproof?
1. What does a materials scientist do?
2. What is the piezoelectric effect?
3. Why could piezoelectric crystals be useful for powering wearable electronics?
4. Explain why a sensor that constantly monitors the body might be better than one that takes measurements just once.
5. What are oxide materials?
6. Name two different forms of silicon dioxide.
7. Why would it be helpful to monitor how much UV radiation hits a person’s skin?
8. What are “microtectonics”?
9. List three types of measurements that John Rogers’s electronics can take so far.
10. Why would scientists or doctors want some electronics to dissolve over time?
1. Besides the uses in the article, imagine some other ways stretchy or sticky electronic devices might help doctors and patients. Describe three you’d like to see — and why.
2. How do you think wearable electronics could help scientists study animals or the environment?
1. Imagine a sensor that measured blood sugar in the body just once. It gave a value of 99 milligrams per deciliter of blood, which is healthy and a rather ideal number. But the body’s blood-sugar values vary dramatically throughout the day (particularly after meals) and during illness. Now, imagine a sensor instead could measure blood-sugar values all day long, reporting the values wirelessly every hour. Suppose it showed that values had varied over a five hour period, climbing from 99 to 130 and 403, then falling to 209 and eventually 51. What was the five-hour average, based on these numbers? And how does that compare to the original 99 mg/dl value? In other words, did it come close to the ideal, and how far did the highest and lowest values deviate (at times), on a percent basis, from that ideal number? Show your work.
Register to access:
An error occurred. Please try again.
Already Registered? Enter your e-mail address above.